How To Determine Odds In Betting
If you were to calculate odds, 10/1 for instance, the formula would be 1 / (10+1) = 0.09. This means that at 10/1 odds, your probability of winning is 9%. If you had fractional odds of 16/3, to provide another example, you could put that into the existing formula and have 3 / (16+3).
- Appendices
- Miscellaneous
- External Links
UFC Betting Info on Odds Shark. The UFC has come a long way since the 1990s when Senator John McCain led a campaign to ban the sport. Luckily for fighters and fans alike, UFC, now partnered with ESPN, has become mainstream and is a popular social sport for friends to gather and watch the fights. Jan 31, 2021 Betting Odds Favourable Tips. Tottenham to win @ 2.25. It goes without saying that Son’s output on Sunday will determine how many points Tottenham will take away from the Amex Stadium. With the above example, let’s calculate the odds of betting the underdog with only $10, instead of $100. Using the +260 odds on the Packers, a $10 bet would payout $26 in profits, plus the return of your original $10 bet for a total of $36 back in your pocket. To calculate winnings on fractional odds, multiply your bet by the top number (numerator), then divide the result by the bottom (denominator). So a $10 bet at 5/2 odds is (10. 5) / 2, which equals $25. A $10 bet at 2/5 odds is (10. 2) / 5, which is $4. What is Implied Probability?
Negative Swings
Over the years I have come across very talented card counters, all of who practiced extensively. Despite these steps they are still unsuccessful players. So the question is why is this? The short answer is that when even the most prepared player doesn't understand the statistics variance they don't size their bet properly to their bankroll. Often times over betting their bankroll. As expected this leads to disastrous results. Here we will go over a few things to consider when determining a blackjack betting strategy. A player can then test their strategies at the 888casino's blackjack page. The statistical swings in blackjack are pretty wide; losing periods can extend beyond what a typical player would consider reasonable, so testing strategy is a necessity.
When playing for any length of time, it is certain that they will experience extended periods of heavy losses. Many will get overly discouraged and leave the business all together. To give you an idea of the variance involved if a player were to play a game where they would have a 2% advantage ( extremely optimistic) over the casino, after 2,500 hands of play there is a 20% chance of you being negative.
In real world examples I was once playing solo and after one week of play and 1,600 hands I was down $15,000. The following week I made $16,000 in 1,200 hands. The swings can be pretty violent so it's vital that you have enough of a bankroll to withstand the negative ones.
Earnings Versus Risk
There is a trade off in risk versus earnings for a static bankroll. Consider two players A and B. Player A decides to bet from $1 to $10 dollars. The chance of Player A losing $10,000 is very low. The actual odds are somewhere in the neighborhood of 1 in 2000. The win rate is approximately $6 per hour.
Player B is a very aggressive player and bets from $100 to $1000. The approximate likely hood of Player B going broke is about 4 in 10 (a winning player never has more than a 50% risk of ruin). However, the win rate for Player B is around $600 per hour. The player or team must determine their threshold for earning versus risk.
3% and 5% Elements of Ruin
Aggressiveness varies between teams. The MIT Blackjack Teams played to a 2-3% Risk of ruin and some of the more aggressive teams like the legendary Al Francesco. Big Player teams played to a 5% risk or ruin. This gives the player a failure rate of 1 chance in 20. A common method for teams is to reduce the unit size by 50% when the bankroll has been halved. With this approach the player sets their risk of ruin to 1 chance in 300.
Earning Expectations
Several of the most respected casino gaming authors have estimated the earning expectations of professional counters to be at least 3%. But through my own playing and conversations with other player's (which easily includes a million hands), we have concluded that a win rate of 1.5% over the long term is a reasonable expectations.
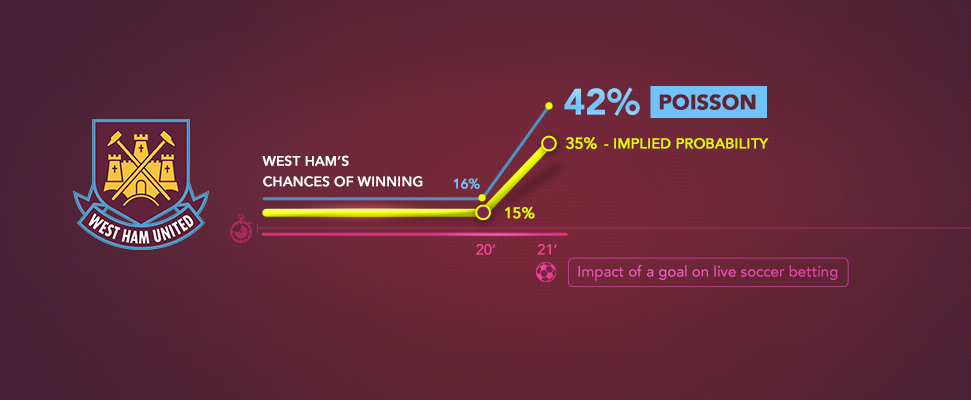
There are a few reasons for the disparity. The first is that many of the authors use computer simulations as a basis for their assertions. No player can play as accurate as a computer; they are bound to make periodic errors. There are several on the fly calculations that the player has to make. Keeping track of cards, adjusting for ace rich or ace poor decks and true count conversions are some of those calculations. This also has to be done with the pit bosses lurking and making the player nervous. With all this to consider, errors are just part of the game. All players make errors, the really good ones make very few.
Even when playing perfectly, it is still not possible to execute the right play. You have to occasionally lay cover plays and some strategy moves have to be avoided because they draw to much attention. For instance splitting 10s is an instant attention getter from the pit.
Lastly, some dealers do cheat. One casino in particular in downtown Las Vegas has a deep reputation for cheating. I don't think that this is a big enough problem to lower the counters edge.
These are a few things the player has to consider when determining their betting strategy. Each strategy is different and the great players are able to mix and match some of the few main pieces and coming up with a few of their original ideas. When the right balance is reached it can be a very profitable endeavor.
Written by: Dustin Jermalowicz
When it comes to gambling, you won’t be able to find two more essential concepts than odds and probability. They are what makes the entire thing work. Odds are used to calculate both the payout a gambler can expect to receive from a winning wager and the implied odds of an outcome happening. Probability is just the likelihood that a given result will occur.
One essential concept to remember is that while probability and odds are both related and may seem very similar, they aren’t exactly the same thing. Probability represents the likelihood that something will happen.
It is calculated by dividing the number of wanted results by the total number of possible outcomes. Odds, on the other hand, present a ratio of wanted results to unwanted outcomes.
There are three primary ways of expressing odds. They are decimal, fractional, and moneyline (or American). No matter what odds format you use, these three types of odds all represent the same thing.
In fact, it is easy to convert one format to another, as you will see further down this guide.
Beyond governing how the entire world of sports betting works, odds play a vital role in helping a sports bettor decide if a bet is worth placing or not. All odds carry with them an implied probability, which we then compare to the real probability to determine whether a wager possesses positive value or not. A rule to live by in the gambling world is only to place bets with positive value.
For ExampleLet’s say we are gambling on the outcome of a single coin flip. Because there are only two sides of a coin, we know that each result has a 50% probability of occurring. For the sake of this example, we are betting tails. We can calculate this like so:
Our desired outcome is for the coin to land on tails, so there is one desired outcome.
We divide the amount of desired outcomes by the total amount of outcomes possible, then multiply the result by one hundred to get the probability. The formula looks like this:
1/2 = 0.5 X 100 = 50%
Now that we know the probability, let’s look at the odds being offered on this bet. For some reason, the odds for heads are set at -300, while tails are +260. This would be a very odd occurrence for such a bet but bear with me. Now we must calculate the implied probability of both lines being offered and determine which bet contains the most value.
First, we will solve the implied probability for heads. I find it easiest to convert the moneyline value to decimal odds before converting to a percentage:
Now we take our decimal odds and convert them to a percentage:
This means the implied odds are a much higher percentage than the actual 50% probability that we already calculated. A bet on heads here would be a terrible decision with a negative value.
Now we will solve for tails:
In this instance, the probability of tails landing far outweighs the implied probability determined by the odds being offered. This is a high-value bet.
Calculating the real probability and comparing that number to the implied probability set by the odds is the primary strategy with which one should approach every bet.
Important:Understanding how to calculate betting odds and find value bets is essential to your long-term success in gambling.
In this guide, we will show you how to convert any format of odds to any other, as well as how to find the implied odds from any type of odds.
Types of Odds Formats
Decimal
Decimal odds are the favorite way to express betting lines in Europe. They are the most straightforward method of communicating odds. The decimal value is the amount that will be returned per each dollar bet. What makes this system particularly helpful is that both the amount staked and the winnings are included.
So let’s say we made a $10 bet at 3.5 odds. Our total return for winning that wager would be $35. $25 is the profit, with the other $10 being the return of our stake.
Fractional
Fractional odds are most commonly found at racetracks or for futures bets when there are entire pools of participants that can possibly win. This format expresses odds in the form of fractions such as 4/1, which would be pronounced “four-to-one.” Four-to-one odds means that you will earn $4 for every $1 that you stake.
Sometimes the fractions will be less straightforward. You may see numbers like 9/2, for example. To calculate the return on a 9/2 bet, let’s pretend that we bet $20 at 9/2 odds for a horse race.
Unlike decimals, fractional odds provide the total payout. They calculate the winnings only. To determine the total amount that you will receive for a winning bet, simply solve the equation above and add $20 to the total. So the formula looks like this:
Moneyline/American
The moneyline system of presenting odds utilizes negative and positive three-digit values to represent which bets are favored or underdogs. A positive number means that a play is considered the underdog. The quantity after the “+” is the amount that will be won for every $100 bet.
On the other end of the spectrum, favorites are displayed with a negative value such as -350. This means that you must bet $350 to win $100. Moneyline odds only calculate the amount potentially won on a bet, and not the total payout.
Calculating Odds
To learn how to calculate odds, let’s make things a bit more interesting with a switch from a coin toss to a roll of a six-sided die. The wager that we are making is that the die will land on 3. In this example, we are looking at one desired outcome. If there are six possible outcomes on a roll of the die, and only one outcome is desirable, that means there are five undesirable results.
How Do Betting Companies Calculate Odds
Because we are calculating the odds, not the probability, we are expressing a ratio of desirable results to undesirable results. In this example, the ratio would look like this:
That means there’s one chance that we will win versus five that we will not. It is important to keep in mind that we are not calculating how likely we are to win, only the ratio of good results to bad.
Now we can calculate the odds against us winning, as well as the odds in favor of a win. To calculate the odds in favor, simply divide the one possible desired outcome by the total outcomes possible.
Conversely, the odds against our win can be solved the same way:
Converting Probability to Odds
You may want to calculate an odds ratio based on a particular probability. In order to solve this equation, we will need to express the probability as a fraction. Using the same six-sided die from before, the possibility of our number landing formatted as a fraction is 1/6.
Next, just subtract the numerator from the denominator:
The answer once again gives us the number of unwanted possible results. Now we just present the odds in ratio form, bringing us to 1:5 odds.
To solve for probability given an odds ratio, we merely reverse the equation. First, we put our odds ratio in fraction form:
Add the numerator and denominator together, which will give us the total number of potential results:
Last, just put the number of wanted outcomes over the total outcomes possible, and we’ve got our probability again!
Converting Odds
There are numerous odds calculators available online that are probably faster to use, but it’s still best that you understand the formulas for converting different odds types to other formats. Below are all of the equations required to transform any kind of odds to any other arrangement.
How To Determine Odds In Betting
The odds always stay the same; they are just represented differently. At times, being able to convert formats can be extremely helpful, especially when switching to decimals when solving for implied probability.
Moneyline to Decimal
To convert positive moneyline odds, the equation is:
(Moneyline odds/100) + 1 = Decimal odds
To convert negative moneyline odds, the equation is:
(100/Moneyline odds) + 1 = Decimal odds
Moneyline to Fractional
To convert positive moneyline odds, the equation is:
(Moneyline odds/100) = Fractional Odds
To convert negative moneyline odds, the equation is:
-100/Moneyline odds = Fractional Odds
Fractional to Decimal
(Numerator/Denominator) + 1 = decimal odds
Fractional to Moneyline
(Numerator/Denominator)
If the result is greater than or equal to 1:
100 X (Answer) = Moneyline odds
If the result is less than 1:
-100/(Answer) = Moneyline odds
Decimal to Fractional
Decimal odds – 1 = X
Put X over 1
How To Read Betting Odds
Example:
- 3.5 – 1 = 2.5
- 2.5/1 = 5/2
- 3.5 decimal odds = 5/2 fractional
Decimal to Moneyline
If decimal odds are greater than 2:
100 X (decimal odds – 1) = Moneyline odds
If decimal odds are less than 2:
-100/(decimal odds -1) = Moneyline odds
Calculating Implied Probability
To make use of our calculations solving for real probability, we must also determine the implied probability. Implied probability converts odds into a percentage.
Note:
That percentage can then be compared to the actual likelihood of an event happening, which allows for intelligent decision making.
In the early coin toss example, we converted our odds from moneyline to decimal before solving for the implied probability. This is not necessary but is often the easiest way to complete the calculation.
From Decimal Odds
Finding implied probability from decimal odds is extremely easy. Let’s say the decimal odds are 2.5.
- 1/2.5 = 0.4
- 0.4 X 100 = 40% Implied Probability
From Moneyline Odds
Calculating implied probability for a -150 favored moneyline bet:
- (- (-150)/((-(-150)) + 100 =
- 150/(150 + 100) = 150/250 = 0.6
- 0.6 X 100 = 60% Implied Probability
Calculating implied probability for an +250 underdog moneyline bet:
- 100/(250 + 100)
- 100/350 = 0.2857
- 0.2857 X 100 = 28.57% Implied Probability
From Fractional Odds
Denominator/(denominator + numerator) X 100
- Calculate the implied probability of 15/2 odds.
- 2/(2 + 15) X 100 = 2/17 X 100 =
- 0.12 X 100 = 12 % Implied Probability
In Conclusion
Understanding what odds and probabilities are, and being able to calculate both, are fundamental skills that anyone aspiring to find any success in sports gambling must possess. The two concepts are closely related and always intertwined, but they are not the same thing.
Odds are represented in ratios of wanted results to unwanted results, while probability is a calculation of wanted outcomes divided by all possible results. Whatever number that calculation produces is the percentage of likelihood that the outcome we want will occur.
Recommended Reading:To judge whether a bet is worth making or not, calculate both the real probability and the implied probability given by the odds being offered. If the actual likelihood is higher than what’s being suggested by the odds, that bet possesses value and should be wagered on. However, if the implied probability is higher, the gamble has a negative value and should be avoided.
Some of these concepts may seem confusing now, but the more you focus on value and calculating odds and probabilities, the easier betting becomes. No longer will you fall for suckers bets offering negative value, nor will you merely make picks based on who you think should win.
The sooner your betting habits become all about identifying valuable odds and betting accordingly, the sooner you’ll see your bankroll start increasing. And that entire process begins with calculating betting odds, so you’ve come to the right place.